Triangle can be formed if

OBJECTIVE
To verify that triangle can be drawn if the sum of lengths of any two sides is greater than the third side.

PREREQUISITE KNOWLEDGE
Familiarity with a triangle and its parts.

MATERIALS REQUIRED
1. A scale
2. A gluestick
3 .Paper Sheet
4. Three Sets  of broom sticks of following measurements

Set-1. 5cm,7cm,11cm
Set-2. 5cm,7cm,14cm
Set-3. 5cm,7cm,12cm

PROCEDURE
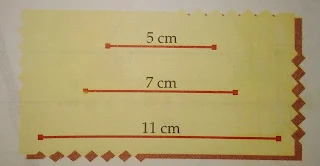
1.Take  set-1 of  broom sticks 
      ( 5cm,7 cm,11cm)   Image 1

          
                                              Image1


2.Try to make a triangle using broom sticks of  Set-1 (Image 2)      
          
                                              Image 2

3.Take other sets ( Set -2,Set-3) of broom sticks  and try to form triangle,we observe that 
forming of  triangle is not possible. (Image3 & Image4)   

           
                                                Image 3

           
                                                 Image 4

OBSERVATION
For set-1

       5+7 >11
       5+11>7
       7+11>5
Triangle can be formed (Image 1)

For set-2

      7+14>5
      5+14>7
      5+7<14       (Not satisfied)
So,triangle can not be formed

For set-3

      7+12>5
      5+12>7
      5+7=12       (Not satisfied)

So,triangle can not be formed

CONCLUSION

So, It is verified that a triangle can be drawn only if the sum of lengths of any two sides 
is greater than the third side.


Comments

Post a Comment

Popular posts from this blog

ज़मीन पर बैठकर खाना खाने के लाभ

ज़मीन पर बैठकर खाना खाने के लाभ What's app message प्राचीनकाल से चली आ रही ज़मीन में खाने की परंपरा को आज लोग नकारने लगे हैं या यूं कहें कि ज़मीन पर बैठकर भोजन करने में वे अब शर्मिंदगी महसूस करने लगे हैं। कुर्सी टेबल पर बैठकर खाना खाना सभ्य और ज़मीन पर बैठकर खाना खाने की परंपरा को असभ्य मानकर अस्वीकार करने लगे हैं। जमीन पर बैठ कर खाने से वज़न कम होता है, पाचन क्रिया ठीक रहती है, दिल स्वस्थ रहता और दिमाग़ तनाव रहित रहता है। ज़मीन पर पर बैठकर खाना खाते समय हम लोग सुखासन की स्थिति में होते हैं, यह पद्मासन का ही एक प्रकार है जो हमारे स्वास्थ्य के लिए बहुत फ़ायदेमंद है। आइए ज़मीन पर बैठकर भोजन करने के फ़ायदों के बारे में जानें। 1. पाचन क्रिया ठीक रहती है ज़मीन पर बैठकर खाना खाते समय हम लोग सुखासन की मुद्रा में बैठते हैं। इस योग मुद्रा में बैठकर खाना खाने से खाना सही तरह से पच जाता है। ज़मीन पर बैठकर भोजन करते समय निवाला उठाते वक़्त आगे की ओर झुकते हैं और फिर निवाला निगलने के बाद पहले वाली पोजिशन में आना जाते हैं। ऐसा बार-बार करने से पेट की मांशपेशियां एक्टिव हो जाती हैं और खाना तेज़ी से पचन...
Read more

NEW NORMAL

Image
Normalcy everyone like but every day new challenges we have to face and we have to adopt some new rules ,regulations ,norms  with the hope that these new rules will bring positive changes in the coming life. There are  always difficulties  before adopting new norms, rules but ultimately we adopt and these new changes become normal, as usual. There are people who do not welcome new things enthusiastically but circumstances force them to accept new changes. Buses, Trains ,Metros, Aeroplanes…etc   all are normal now a days but they   also faced difficulties in making their place in the society. When computer   was introduced then nobody liked it because there was common opinion that it would turn many people unemployed, but   it is impossible to think about present life without computers. Now, This has also become normal. Like lock down was introduced to prevent   corona pandemic, first it was very difficult to remain always inside the home ...
Read more

before the exam.

Image
One should avoid the following things before the exam. Don't think that you had forgot everything. Don't try to prepare new chapter before revising the already prepared chapters. Don't awake whole night. Don't eat heavy. Don't read anything just before the exam. Don't repent that you couldn't study well Don't start writing before reading the paper twice. Don't use to many pens for writing. Don't put wrong question number. Don't put your pens and other things anywhere otherwise how will you get them before the exam.
Read more